Notices
 

Browse & Filter

For page specific messages
For page author info
Enter a comma separated list of user names.
2651 records found.
Operations
Selected 20 rows in this page.  
Title+Summary Name Datesort ascending

Testing Copy paste from Browsing results

Node id: 5374page
 kapoor's picture 22-04-17 09:04:25 n

[2013EM/HMW-06]

Node id: 5380page
 AK-47's picture 22-04-17 09:04:45 n

[2013EM/HMW-04]

Node id: 5378page
 AK-47's picture 22-04-17 09:04:12 n

[2013EM/HMW-03]

Node id: 5377page
 AK-47's picture 22-04-17 09:04:54 n

[2013EM/HMW-02]

Node id: 5376page
 AK-47's picture 22-04-17 09:04:01 n

[2013EM/HMW-01]

Node id: 5375page
 AK-47's picture 22-04-17 08:04:36 n

QM-22 WKB Approximation

Node id: 764page

Exercises

This set has practice problems on WKB approximation for bound state energy levels.

qm-exe-22001

 kapoor's picture 22-04-17 06:04:24 n

[QUE/CM-02037] An Uphill Problem

Node id: 3105page

Watch the yoututbe video. 

https://www.youtube.com/watch?v=SUjY0sdtGus

  1. Describe what you see in the video.
  2. Set up the Lagrangian for (a) cylinder, (b) a double cone, rolling on the rails.
  3. Solve the equations of motion and show that the cylinder climbs down while the double  cone will climb up  the rails.

 

 

 kapoor's picture 22-04-16 13:04:45 n

[QUE/CM-09001]

Node id: 2207page


Show that the kinetic energy of a uniform rod of mass \(m\) is
\[ T  = \frac{1}{6}m(\vec{u}.\vec{u} + \vec{u}.\vec{v}+\vec{v}.\vec{v} )\]
where \(\vec{u}\) and \(\vec{v}\) are the velocities of the two ends.

Woodhouse

 kapoor's picture 22-04-16 11:04:00 n

[QUE/CM-07010] Small Ocillations and He molecule

Node id: 2238page

Assuming that the van der Waals force between two He  atoms is described by Lenard Jones potential
\[ V(r) = \epsilon_0 \Big( \frac{r_0^{12}}{r^{12}}- \frac{r^6_0}{r^6}\Big)\]
and that  \(\epsilon_0=1.42\times10^{15} \text{erg},\qquad r_0= 2.87\times10^{-8}\text{cm}\).

Determine

  1. the force constant for small  oscillations
  2. the fequency of oscillations for the vibrational motion of a bound pair of He atoms.
  3. Using the  approximate results obtained above and quanum mechanical expression for vibrational energy of He molecule, determine whether or not two He atoms canform a molecule He\(_2\). ( Note that these results are only approximate  (and can in fact lead to the worng conclusion!) but nevetheless they are instructive. Can you guess why the result might be wrong?

Atlee Jackson*   

 

 kapoor's picture 22-04-16 11:04:54 n

[QUE/CM-07011] Small Oscillations of Oxygen Molecule

Node id: 2239page

Assuming that the van der Waals force between two oxygen  atoms is described by Lenard Jones potential \[ V(r) = \epsilon_0 \Big( \frac{r_0^{12}}{r^{12}}- \frac{r^6_0}{r^6}\Big)\]
and that  \(\epsilon_0=16.4\times10^{15}\) \text{erg}, r_0= 3.88\times10^{-8}\text{cm}\) determine

  1. the force constant for small  oscillations
  2. the fequency of oscillations for the vibrational motion of a bound pair of He atoms.
  3. Using the  approximate results obtained comote the numerical value of the vibration of oxygen molecule. How do explain the differences between your answer and the experimenl value \(\nu=6.45\times10^{13}\).

Atlee Jackson*   

 

 kapoor's picture 22-04-16 11:04:54 n

[QUE/CM-08001]

Node id: 2348page

A mass point $M$ is moving under gravity along a rod, which rotates about the vertical axis passng through the fixed end of the rod. The angle between the rod and the vertical axis remains constant equal to $\alpha$.

  1. Set up the Newtonian equations of motion
  2. Set up the Lagrangian and obtain the equations of motion.
  3. Discuss the motion using the generalized coordinates in (b).

  [SDG]

 kapoor's picture 22-04-16 11:04:31 n

[QUE/CM-08003]

Node id: 2350page

A perfectly smooth horizontal disk is rotating with an angular velocity $\omega$ about a vertical axis passing through its center. A person on the disk at a distance $R$ from the origin gives a perfectly smooth coin (negligible size)pf mass $m$ a push towards the origin. This push gives it an initial velocity $V$ relative to the disk. Show that the motion for a time $t$, which is such that $(\omega t)^2$ is negligible, appears to the person on the disk to be a  parabola, and give the equation of the parabola.

      [Lim 1098]

 kapoor's picture 22-04-16 11:04:46 n

[QU/CM-08002]

Node id: 2349page

Consider a hoop of radius $a$ in a vertical plane  with angular velocity $\omega$ about the vertical diameter. Consider a bead of mass $m$ which slides without friction on the hoop as indicated in figure.
Under what condition will the equilibrium of the bead at  $\theta=0$ be stable?

  1. Find another value of $\theta$ for which, in certain circumstances, the bead will be in stable equlibrium. Indicate the values of $\omega$ for which this stable equilibrium takes place.
  2. Explain your answer with the aid of appropriate graphs of the potential energy versus $\theta$ as measured in the rotating frame.                                                                      [Lim 1097]

Problem-CM-08002

kapoor's picture 22-04-16 11:04:23 n

[QUE/CM-07006]

Node id: 2356page

A thin uniform bar of mass $m$ and length \(\frac{3L}{2}\) is suspended by a spring of length \(L\) and force constant \(k\) and negligible mass. Find the normal frequencies and normal modes of small oscillations in a plane.

 kapoor's picture 22-04-16 11:04:19 n

QUE/CM-07009] A small oscillation problem in two dimensions

Node id: 2355page

 Consider a particle of mass \(m\) moving in two dimensions in a potential \[ V(x,y) =   
     \frac{k}{2}(5 x^2 + 2 x y + 5 y^2)\]

  1.   At what point \((x_0,y_0)\) is the particle in stable  equilibrium?
  2.   Give the Lagrangian appropriate for small oscillations about this equilibrium   position.
  3.   Find the normal frequencies of vibration in (b).
  4. Write the Lagrangian in terms of normal coordinates and verify that it takes the form \[\mathscr{L} = \frac{1}{2}(\dot{Q}_1^2 + \dot{Q}_2^2) -\frac{1}{2}( Q_1^2 + Q_2^2)\]
kapoor's picture 22-04-16 11:04:09 n

[QUE/CM-08004]

Node id: 2708page

Let A be a real  antisymmetric matrix.

  1. Show that $|I\,+\,A| $ is nonsingular.
  2. Show that $$ B\ =\ (I\,+\,A)(I\,-\,A)^{-1} $$

an orthogonal matrix.

 kapoor's picture 22-04-16 11:04:00 n

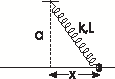
[QUE/CM-07001]

Node id: 2351page

 

The figure shows a body of mass $m$ constrained to move in a
horizontal line under the influence of a massless spring of
natural length \(L\) and spring constant $k$. Find the equilibrium
points for the following three cases.

  1. Case \(a=L\): When the mass is at $x=0$, the spring is at its equilibrium length.
  2. Case \(a>L\): When $x=0$, the spring is stretched.
  3. Case \(a < L\): When $x=0$, the spring is compressed.
  4. Find the period of small oscillations in case (a).
kapoor's picture 22-04-16 11:04:02 n

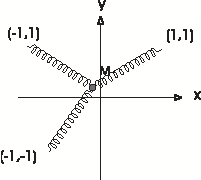
[QUE/CM-07002] Three Springs Problem

Node id: 2352page
Three massless springs of natural length $\surd 2$ and spring constant
      $k$ are attached to a point particle of mass $m$ and to the fixed
      points $(-1,1), (1,1)$ and $(1,1)$ as shown in the Fig. 1. The point
      mass is allowed to move in the $(x,y)$ plane only.

  1. Write the Lagrangian for the system.
  2. Is there an equilibrium position for the point mass? Where is it?
  3. Give the Lagrangian appropriate for small oscillations.
  4. Find the frequencies and normal coordinates of small oscillations.
  5. Sketch the normal modes.
kapoor's picture 22-04-16 11:04:51 n

[QUE/CM-07007] Double Pendulum

Node id: 2353page
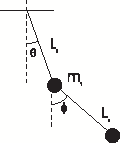Double Pendulum Set up the Lagrangian for double pendulum consisting of two masses  $m_1$ and $m_2$ connected to light bars of lengths $l_1$ and $l_2$ as shown in figure. Find the frequencies of small oscillations for the case of equal masses, $m_1=m_2$ and equal lengths $l_1=l_2.$
 
kapoor's picture 22-04-16 11:04:32 n

Pages

 
X