|
|
[2019EM/HMW-06]Node id: 5368pageElectrodynamics Oct 17, 2018
Tutorial-V (VI)
- A cylindrical resistor of length \(\ell\), radius \(a\) and resistivity $\varrho$ carrying a current \(i\).
- Show that the Poynting vector $\vec{S}$ at the surface of the resistor is everywhere directed to the normal to the surface, as shown,
- Show the rate $\rho$ at which energy flows into the resistor through its cylindrical surface, calculated by integrating the Poynting vector over this surface, is equal to the rate at which Joule heat is produced, i.e. \begin{equation*} \int\vec{S}\cdot\vec{dA}=i^2R \end{equation*}
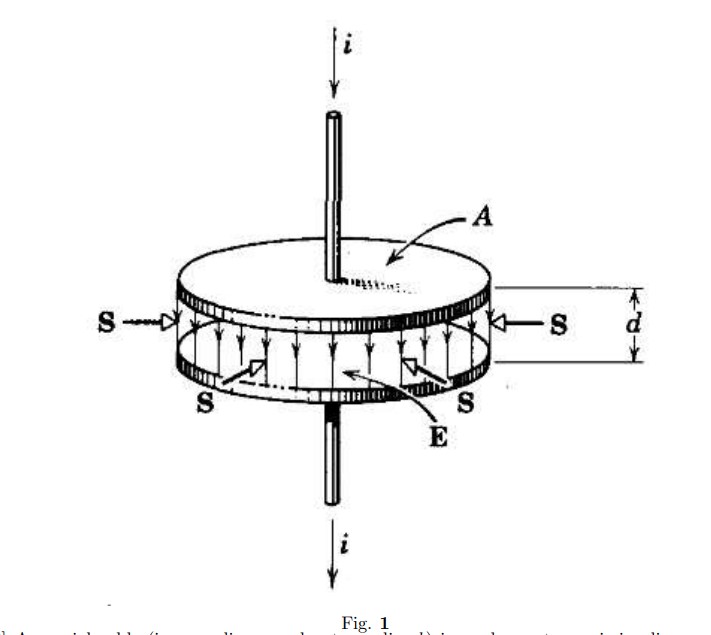
- Figure 1 shows a parallel-plate capacitor being charged,
- Show that the Poynting vector \(S\) points everywhere radially into the cylindrical volume.
- Show that the rate \(P\) at which energy flows into this volume, calculated by integrating the Poynting vector over the cylindrical boundary of this volume, is equal to the rate at which the stored electrostatic energy increases; that is, that \[\int \vec{S}\cdot\vec{dA} = A d \frac{1}{2}\frac{d}{dt}(\epsilon \vec{E}^2)\],where \(Ad\) is the volume of the capacitor and \(\frac{1}{2} \epsilon_0\vec{E}^2\) is the energy density for all points within that volume. This analysis shows that, according to the Poynting vector point of view, the energy stored in a capacitor does not enter it through the wires but through the space around the wires and the plates. \{Hint: To find \(S\) we must first find \(B\), which is the magnetic field setup by the displacement current during the charging process; Ignore fringing of the lines of \(E\).)\}
- A coaxial cable (inner radius \(a\) and outer radius \(b\)) is used as a transmission line between a battery $\epsilon$ and a resistor \(R\),
- [(a)] Calculate $\vec{E}, \vec{B}$ for $a < r < b$
- [(b)] Calculate Poynting vector S for $a< r < b.$
- [(c)] By suitably integrating the Poynting vector, Show that the total power flowing across the annular cross section $a<r<b$ is="" $\epsilon^{2}="" r$.="" this="" reasonable="" ?="" <="" li="">
- [(d)] Show that the direction of $\vec{S}$ is always away from the battery to resistor, no matter which way is the battery connected.
|

|
22-04-25 19:04:10 |
n |
|
|
[2019EM/HMW-05]Node id: 5367pageElectrodynamics Apr 14, 2018
Tutorial-V
- A cylindrical resistor of length \(\ell\), radius \(a\) and resistivity $\varrho$ is carrying a current \(i\).
- Show that the Poynting vector $\vec{S}$ at the surface of the resistor is everywhere directed to the normal to the curved surface.
- Show the rate $\rho$ at which energy flows into the resistor through its cylindrical surface, calculated by integrating the Poynting vector over this surface, is equal to the rate at which Joule heat is produced, i.e. \begin{equation*} \int\vec{S}\cdot\vec{dA}=i^2R \end{equation*}
- A long coaxial cable consists of two concentric conductors of radii \(a,b\) and length \(\ell\), see \Figref{Cable-Self-Ind}. The inner conductor is assumed to be a thin cylindrical shell. The conductors carry currents in opposite directions. Calculate self inductance of this cable in two ways:
- Using the definition of self inductance
- Using energy considerations.
- \Figref{Capacitor} shows a parallel-plate capacitor being charged,
- Show that the Poynting vector \(S\) points everywhere radially into the cylindrical volume.
- Show that the rate \(P\) at which energy flows into this volume, calculated by integrating the Poynting vector over the cylindrical boundary of this volume, is equal to the rate at which the stored electrostatic energy increases; that is, that \[\int \vec{S}\cdot\vec{dA} = A d \frac{1}{2}\frac{d}{dt}(\epsilon \vec{E}^2)\],where \(Ad\) is the volume of the capacitor and \(\frac{1}{2} \epsilon_0\vec{E}^2\) is the energy density for all points within that volume. This analysis shows that, according to the Poynting vector point of view, the energy stored in a capacitor does not enter it through the wires but through the space around the wires and the plates. \{Hint: To find \(S\) we must first find \(B\), which is the magnetic field setup by the displacement current during the charging process; Ignore fringing of the lines of \(E\).)\}
- What happens when the current is taken to flow in the opposite direction?
- The Sun delvers about 1000 W/m\(^2\) of energy to earth's surface.
- Compute the power incident on a roof of dimension 5.0m\(\times\)8.0 m.
- Determine the radiation pressure and radiation force on the roof,assuming that the roof is a perfect absorber. \end{AlphaList1} You may assume that the Sun's rays are incident at 90\(^o\) on the roof. % Serway and Jerwett p315
|

|
22-04-25 19:04:57 |
n |
|
|
[2019EM/HMW-04]Node id: 5366pageElectrodynamics March 26, 2019
Tutorial-IV
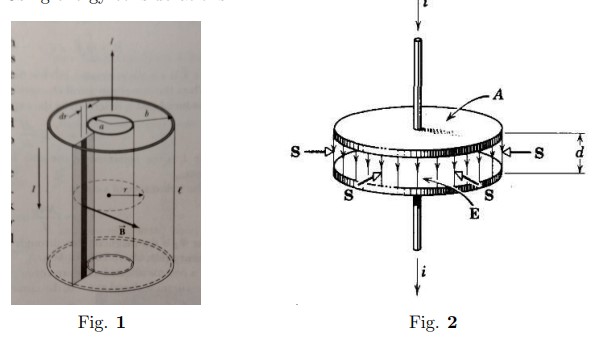
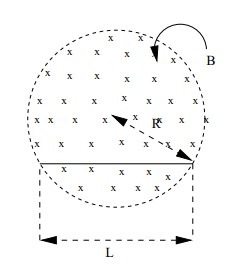
- A uniform magnetic field $\vec{B}$ fills a cylindrical volume of radius $R$ and a metal rod of length $L$ is placed in it as shown in the figure.If the magnitude $B$ is changing at the rate $\frac{dB}{dt}$ show that the emf that is produced by the changing magnetic field and that acts between the ends of the rod is given by $${\cal E} = \frac{dB}{dt}\frac{L}{2}\sqrt{R^2-\frac{L^2}{2}}$$
- Two identical coils each having radius $R$ and $n$- turns are kept parallel and with a distance $d$ between the two.
- Find an expression for the magnetic field at a point on the common axis of the coils and at a distance $x$ from the mid-point between the coils.
- Show that if the separation of the coils is equal to $R$, the first and the second derivatives of $B$ w.r.t. $x$ vanish at the mid point. This produces nearly constant magnetic field near the mid point, WHY?
- For $R=5.0$cm, $I=50$amp,and 300 turn coils, plot the magnetic field as a function of $x$ in the range $x=-5$cm to $x=5$cm.
.
- A wooden cylinder of mass $m=0.5$kg, radius $R=3$cm, length $\ell=10$cm, is placed on an inclined plane. It has 10 turns of wire wrapped around it longitudinally so that the plane of the wire contains the axis of the cylinder and is parallel to the inclined plane, see . Assuming no friction, what is the current that will prevent the cylinder from rolling down the inclined plane in presence of a uniform magnetic field of 0.5T?. Describe what happens if the block is a rectangular instead of a cylindrical one? What will be the current that will prevent the block from moving down the plane?
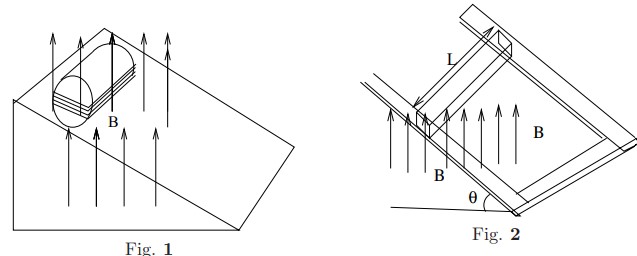
- A square wire of length $L$, mass $m$, and resistance $R$ slides without friction down parallel rails of negligible resistance, as in \Figref{em-fig-015}. The rails are connected to each other at the bottom by a resistanceless rail parallel to the wire so that the wire and rails form a closed rectangular conducting loop. The plane of the rails makes an angle $\theta$ with the horizontal, and a uniform vertical magnetic field $\vec{B}$ exists in the region.
- Show that the wire acquires a steady state velocity of magnitude $$v= \frac{mgR\sin\theta}{B^2 L^2\cos^2\theta}$$
- Show that the above result is consistent with conservation of energy.
- What changes will be necessary in the above results, if the direction of magnetic field is reversed?
- A cylindrical shell of radius $R$, height $h$, and carrying a uniform surface charge density $\sigma$, rotates about its own axis with angular velocity $\omega$. Compute the magnetic field produced by the cylinder at a point on the axis
|

|
22-04-25 19:04:47 |
n |
|
|
SampleRankingQuiz Node id: 275page |

|
22-04-25 19:04:37 |
n |
|
|
[QUE/GT-02006]Node id: 3796pageFor permutation group of 3 objects
- Construct the multiplication table;
- Find cosets of the group;
- Verify that the classes of $S_3$ are $$\{e\},\{(1,2),(2,3),(1,3)\},\{(1,2,3),(1,3,2)\}$$
|

|
22-04-25 09:04:07 |
n |
|
|
[QUE/GT-02002]Node id: 3793pageList all the elements of symmetries of a square and construct the
group multiplication table. Use the multiplication table construct the class
multiplication rules.}
Notation:Name the group elements as follows.
- Anticlockwise rotation about \(z\)- axis by angle \(\pi/2\) \(:\to 4_z\).
- Anticlockwise rotation about \(z\)- axis by angle \(\pi\)\(:\to 4_z^2\).
- Anticlockwise rotation about \(z\)- axis by angle \(3\pi/2\)\(:\to 4_z^3\).
- Reflection in a plane perpendicular to \(X\)- axis \(:\to m_x\)
- Reflection in a plane perpendicular to \(Y\)- axis \(:\to m_y\)
- Reflection in plane containing \(Z\)- axis and diagonal 13 \(:\to m_{13}\)
- Reflection in plane containing \(Z\)- axis and diagonal 24 \(:\to m_{24}\)
The classes are given to be\\
\begin{equation*} \begin{array}{ll} C_1=\{e\}.& \\ C_2 =\{4_z^2\} &C_3 =\{4_z,
4_z^3\} \\ C_4=\{m_x, m_y\} &C_5 =\{m_{13}, m_{24}\} \end{array}\end{equation*} |

|
22-04-25 09:04:22 |
n |
|
|
[QUE/VS-10005]Node id: 3786pageGive an example of a \(3\times 3\) matrix \(P\) such that \(P^2= P\).
|

|
22-04-25 09:04:42 |
n |
|
|
[QUE/VS-10006]Node id: 3785pageIn \(C^3\) with scalar product \((x,y)=x^\dagger y\) find the adjoint of operator \(A\) where \(A\) is defined to be
\begin{equation}
A \begin{pmatrix}x_1\\ x_2\\ x_3 \end{pmatrix}
= \begin{pmatrix}x_1+ x_2\\ x_2+x_3 \\ x_3+x_1\end{pmatrix}
\end{equation} |

|
22-04-25 09:04:35 |
n |
|
|
[QUE/VS-10004]Node id: 3784pageGive an example of a positive definite \(3\times 3\) matrix with all matrix elements non zero. |

|
22-04-25 09:04:11 |
n |
|
|
[QUE/VS-10003]Node id: 3783pageConsider the vector space of all polynomials \(p(t)\) of degree less than or 5 with inner product
\[((p,q) = \int_{-1}^1 p(t) q(t) \, t^2\, dt \] Is the operator \[ A p(t) = \frac{ d p(t)} {dt}\]
self adjoint? |

|
22-04-25 09:04:46 |
n |
|
|
[QUE/QCQI-01001]Node id: 3807pageGiven two normalized vectors \(\chi_1\) and \(\chi_2\):
\begin{equation}
\chi_1=\begin{pmatrix}\cos\alpha\\ \sin\alpha\end{pmatrix}\qquad
\chi_2=\begin{pmatrix}\cos\beta\\ \sin\beta\end{pmatrix},
\end{equation}
find conditions on \(\alpha,\beta \) so that \(\chi_1+\chi_2\) may be a normalized vector.
Answer : \(\alpha-\beta=\frac{2\pi}{3}, or \frac{4\pi}{3}\)
Source: W.H. Steeb* |

|
22-04-25 09:04:03 |
n |
|
|
[QUE/VS-10002]Node id: 3782pageThe matrix of a linear operator \(A\) in an orthonormal basis \(\{e_1, e_2,
e_3\}\) is given to be
\begin{equation}
\underline{\sf A} = \begin{pmatrix}
0 & 1 & 0 \\
0 & 0 & 1 \\
1 & 0 & 0
\end{pmatrix}
\end{equation}
Is this operator unitary? How is the basis \(\{f_1, f_2,f_3\}\)
in which the operator is diagonal related to \(\{e_1, e_2,e_3\}\)? |

|
22-04-25 09:04:41 |
n |
|
|
[QUE/GT-02007]Node id: 3797pageFind all left and right cosets of the group of permutations on three objects, $S_3$ taking the subgroup to be
- $H_1=\big\{e, (1,2)\big\}$
- $H_2=\big\{e, (1,3)\big\}$
- $H_3=\big\{e, (2,3)\big\}$
- $H_4=\big\{e, (1,2,3),(1,3,2)\big\}$
|

|
22-04-25 09:04:34 |
n |
|
|
[QUE/GT-02004]Node id: 3795pageThe classes of the permutation group $S_3$ are given to be $$ C_0 =\{e\}, C_1=\{(1,2),(1,3),(2,3)\}, C_2 = \{(1,2,3),(1,3,2)\} $$ Express $C_1^2,C_2^2$ and $C_1 C_2$ in terms of the classes and find the class constants. |

|
22-04-25 09:04:57 |
n |
|
|
[QUE/VS-10001]Node id: 3781pageIn the Hilbert space of square integrable functions \(L^2(-\infty, \infty)\) find adjoint of an operator \(X\) defined by \[ T \psi(x) = \psi(ax+b),\quad a\ne0, \text{ and } a,b\in R \] |

|
22-04-25 09:04:49 |
n |
|
|
[QUE/02003]-GTNode id: 3794pageWhat is the number of irreducible representations of the D-4 group.
Find their dimensions and construct the character table for the group.
You may use the notation and information given below.}
- $\oslash$ Notation for group elements
- Anticlockwise rotation about \(z\)- axis by angle \(\pi/2\) \(:\to 4_z\).
- Anticlockwise rotation about \(z\)- axis by angle \(\pi\)\(:\to 4_z^2\).
- Anticlockwise rotation about \(z\)- axis by angle \(3\pi/2\)\(:\to 4_z^3\).
- Reflection in a plane perpendicular to \(X\)- axis \(:\to m_x\)
- Reflection in a plane perpendicular to \(Y\)- axis \(:\to m_y\)
- Reflection in plane containing \(Z\)- axis and diagonal 13 \(:\to m_{13}\)
- Reflection in plane containing \(Z\)- axis and diagonal 24 \(:\to m_{24}\)
The classes are given to be \begin{equation*} \begin{array}{ll} C_1=\{e\}.& \\
C_2 =\{4_z^2\} &C_3 =\{4_z, 4_z^3\} \\
C_4=\{m_x, m_y\} &C_5 =\{m_{13}, m_{24}\}
\end{array}
You may use class multiplication rules \begin{equation*} \begin{array}{llll}
C_2^2=C_1 & C_2C_3=C_3 & C_2C_4=C_4 & C_2C_5 = C_5\\
C_3^2 = 2C_1+C_2 & C_3C_4= 2C_5 & C_3 C_5 = 2C_4 &\\
C_4^2 =2C_1+2C_2 & C_4 C_5= 2C_3 &&\\
C_5^2= 2C_1+2C_2 &&&
\end{array} \end{equation*}

|

|
22-04-25 08:04:58 |
n |
|
|
[QUE/VS-11002]Node id: 3792pageConsider the operator \[ A p(t) = \frac{d p(t)}{dt}, \quad \text{on } P^3(t).\] Is the subspace \(P^2(t) \) an invariant subspace of the operator \(A\)? Notation: \(P^n(t) \) is space of all polynomials of degree less than or equal to \(n\). |

|
22-04-25 08:04:28 |
n |
|
|
[QUE/VS-01-001] Periodic motionNode id: 3698pageConsider the set of all vectors \(\xi=(\xi_1,\xi_2,\xi_3)\) in \(\C^(3)\) for
which
- \(\xi_1\) is real
- \(\xi=0\)
- \(|\xi_1|> 0\)
- either \(\xi_1\) or \(\xi_2\) equal to zero
- \(\xi_1+\xi_2=0\)
- \(\xi_1+\xi_2=1\)
In which of the above cases do the set of all vectors form \(\xi\) form a vector
space? |

|
22-04-25 08:04:45 |
n |
|
|
[QUE/VS-11001]Node id: 3791pageLet \(A\) be an operator having eigenvalues 4 and 9 and corresponding
eigenvectors are
\begin{equation*}
e_1 =\begin{pmatrix} 1 \\ i \end{pmatrix};\qquad
e_2=\begin{pmatrix}-1\\i\end{pmatrix}\\[-6mm]
\end{equation*} For an arbitrary vector \(f=\begin{pmatrix}\alpha \\ \beta \end{pmatrix}\) find the vectors
- \(A f\)
- \(\sqrt{A} f\)
- \(A^2 f\)
- \(\exp\Big(i\pi A/6 f \Big)\)
Write the matrices for (i) \(\sqrt{A}\), (ii) \(\exp\big(i\pi A/6 f\big)\). |

|
22-04-25 08:04:50 |
n |
|
|
[QUE/GT-02008]Node id: 3798pageFind all subgroups of the group of permutations $S_3$ on three objects.
For each subgroup check if it is an invariant subgroup.
For each class, explicitly verify the result that every invariant subgroup
either contains all elements of the class, or else is disjoint from the class. |

|
22-04-25 08:04:59 |
n |