|
|
[QUE/TH-03001] TH-PROBLEMNode id: 5154pageConsider \(N\) molecules of a gas obeying van der Waals
equation of state given by
\[\left(P+ \frac{a N^2}{V^2}\right)\big(V-Nb\big)
= Nk_B T\]
where \(a\) is a measure of the attractive forces between
the molecules and \(b\) is another constant proportional to
the size of a molecule. The other symbols have their
usual meanings. Show that during an isothermal expansion (Temperature is kept
constant) from volume \(V_1\) to
volume \(V_2\) quasi-statically and reversibly, the work done is
\[ W =-Nk_B T \log\left(\frac{V_2-Nb}{V_1-Nb}\right) + a^2
\Big(\frac{1}{V_1}-\frac{1}{V_2}\Big)\] |

|
22-01-13 18:01:03 |
n |
|
|
[QUE/TH-03002] TH-PROBLEMNode id: 5155pageAn ideal gas is enclosed in a cylindrical volume with a movable piston. It can be taken from a state 1 to state 2 by several processes as shown in \(P-V \) diagram below. It is given that if the walls are adiabatic the system obeys the equation \(P^3 V^5=\text{const}\) The energy of the state in state 1 is given to be 100J. The pressures and volume at for the two states are given as follows. \[P_1=10^5 \,\text{Pa}, V_1=10^{-3}\, \text{m}^3; P_2 =\Big(\frac{10^5}{32}\Big) \,\text{Pa}, V_2= b\times 10^{-3}\, \text{m}^3.\] {\textwidth}{ Several paths taking the system from state 1 to state 2 are shown in figure below.
- AB followed by BC
- AD followed by DC
- Linear path connecting A and C
- Some other path AQC
Your are required to compute the internal energy of the system in state 2 by computing work done by selecting a path from one of the above options. If you choose option (d), some other path, specify the path. Compute the work done along the path chosen and find the internal energy of system in state 2. |

|
22-01-13 18:01:59 |
n |
|
|
[QUE/TH-06006] TH-PROBLEMNode id: 5062page Consider a photon gas in two dimensions at temperature T in area A. Find the energy density $u(\omega)$ as a function of temperature and various physical constants. Show that the total energy is proportional to $T^3$. ( you can assume that the internal degree of freedom is 1. ) |

|
22-01-13 18:01:29 |
n |
|
|
[QUE/SM-03001] SM-PROBLEMNode id: 5065pageConsider a system of $N$ atoms. Assuming that they can exist in two states only. The ground state having energy zero and an excited state having energy $\epsilon$.
- Find the number of micro states with total energy $U$.
- Write an expression for entropy and using Stirling approximation for the factorial $$ \ln (N!) \approx N \ln N - N$$ find the temperature of the system and hence show that $$ U = \frac{N\epsilon}{1+ e^{\epsilon/kT}}$$
- What is fraction of atoms are in the excited state at very large temperature $(T >> kT)$?
|

|
22-01-13 18:01:58 |
n |
|
|
[QUE/TH-06002] TH-PROBLEMNode id: 5161pageConsider a cycle $ABCD$ with perfect gas as the working substance. $AB$ is at constant volume $V_1$ and $CD$ is at constant volume at $V_2$ with $V_2\,>\,V_1$ The parts $BC$ and $DA$ are adiabatic. Calculate the efficiency of this engine in terms of $V_1$ and $V_2$. (Note this is different from Carnot's engine and so we can not draw similar conclusions about the efficiency being maximum) |

|
22-01-13 18:01:03 |
n |
|
|
[QUE/TH-06001] TH-PROBLEMNode id: 5160pageTwo heat reservoirs, each of fixed volume $V$ are at temperatures $T_1$ and $T_2$ ( $T_1\,>\,T_2$) initially. An engine operates between the two till both of them reach the same temperature $T_3$. The specific heat $c_V$ of the reservoirs can be assumed to constant through out. The engine is back to its inital state.
- Show that $$ T_3^2\,\geq\,T_1T_2 $$
- Calculate the maximum work that can be extracted from the two reservoirs. ( Answer in terms of $T_1$ and $T_2$)
|

|
22-01-13 18:01:06 |
n |
|
|
[QUE/TH-06003] TH-PROBLEMNode id: 5162pageShow that if
$$ \left(\frac{\partial U}{\partial V}\right)_T\,=\,0$$
then
$$ \left(\frac{\partial U}{\partial P}\right)_T\,=\,0 $$
with
$$ \left(\frac{\partial P}{\partial V}\right)_T\,\neq\,0$$ |

|
22-01-13 18:01:36 |
n |
|
|
[QUE/TH-07001] TH-PROBLEMNode id: 5165pageThe tension $\tau$ in an elastic rubber band is given by $$ \tau\,=\,aT\left(\frac{L}{L_0(T)}-\,\left(\frac{L_0(T)}{L}\right)^2\right),$$ where $a$ is a constant, $L_0(T)$is the unstretched length at zero tension, and is a function of temperature only.
- Write the first law using the work done when it is elongated and gets a supply of heat. ( Be careful of signs!)
- Use the first law to write $dF$, where $F$ is the free energy of the rubber band.
- Solve for the free energy $F$ and show that $$ F(T,L)\,-\,F(T,L_0(T))\,=\,aT\left(\frac{L^2}{2L_0(T)}\,+\,\frac{L_0(T)^2}{L^2}\,-\,\frac{3L_0(T)}{2}\right)$$ and the entropy $S$ $$ S(T,L)\,-\,S(T,L_0(T))\,=\,a\left(\frac{3L_0}{2}\,-\,\frac{L_0^2}{L}\,-\,\frac{L^2}{2L_0}\right)\,-\,aT\left(\frac{3}{2}\,-\,\frac{2L_0}{L}\,+\,\frac{L^2}{2L_0^2}\right)\frac{dL_0(T)}{dT} $$
- Find the heat $Q$ transferred to the elastic band when it is stretched from $L_0$ to $L$ isothermally.
- Show that $$ \left(\frac{\partial T}{\partial L}\right)_S \,=\,\frac{aTL_0^2}{c_LL^2}\left(-1\,+\,\left(\frac{L}{L_0}\right)^3\,+\,\frac{Ta}{L_0}\frac{dL_0}{dT}\left(2\,+\,\left(\frac{L}{L_0}\right)^3\right)\right)$$ where $$c_L\,=\,\left(\frac{DQ}{\partial T}\right)_L.$$
|

|
22-01-13 18:01:49 |
n |
|
|
[QUE/TH-07002] TH-PROBLEMNode id: 5166pageFind the specific heat $C_H$ of a substance at constant enthalpy and show that it is equal to
$$ C_H\,=\,\frac{C_p}{1\,-\,\frac{T}{V}\times\left(\frac{\partial V}{\partial T}\right)_p} $$
where we are using the standard notation.
|

|
22-01-13 18:01:19 |
n |
|
|
[QUE/TH-08001] TH-PROBLEMNode id: 5167pageThe tension $\tau$ in an elastic rubber band is given by
$$ \tau\,=\,aT\left(\frac{L}{L_0(T)}-\,\left(\frac{L_0(T)}{L}\right)^2\right),$$
where $a$ is a constant, $L_0(T)$is the unstretched length at zero tension, and is a function of temperature only.
(a) Write the first law using the work done when it is elongated and gets a supply of heat. ( Be careful of signs!)
(b) Use the first law to write $dF$, where $F$ is the free energy of the rubber band.
(c) Solve for the free energy $F$ and show that
$$ F(T,L)\,-\,F(T,L_0(T))\,=\,aT\left(\frac{L^2}{2L_0(T)}\,+\,\frac{L_0(T)^2}{L^2}\,-\,\frac{3L_0(T)}{2}\right)$$
and the entropy $S$
$$ S(T,L)\,-\,S(T,L_0(T))\,=\,a\left(\frac{3L_0}{2}\,-\,\frac{L_0^2}{L}\,-\,\frac{L^2}{2L_0}\right)\,-\,aT\left(\frac{3}{2}\,-\,\frac{2L_0}{L}\,+\,\frac{L^2}{2L_0^2}\right)\frac{dL_0(T)}{dT} $$
(d)Find the heat $Q$ transferred to the elastic band when it is stretched from $L_0$ to $L$ isothermally.
(e) Show that
$$ \left(\frac{\partial T}{\partial L}\right)_S \,=\,\frac{aTL_0^2}{c_LL^2}\left(-1\,+\,\left(\frac{L}{L_0}\right)^3\,+\,\frac{Ta}{L_0}\frac{dL_0}{dT}\left(2\,+\,\left(\frac{L}{L_0}\right)^3\right)\right)$$
where
$$c_L\,=\,\left(\frac{DQ}{\partial T}\right)_L.$$
|

|
22-01-13 18:01:27 |
n |
|
|
[QUE/TH-08002] TH-PROBLEMNode id: 5168pageUse Maxwell's relations to find a relation between the specific heats, coefficient of thermal expansion and compressibility and show that
\[C_p= C_V+ \frac{T\alpha^2}{\kappa_T} V\] |

|
22-01-13 18:01:38 |
n |
|
|
[QUE/TH-09001] TH-PROBLEMNode id: 5169pageConsider the following expressions for entropy. Which ones can possibly be a fundamental equation and which ones violate one or more of postulates II,III and IV?
- $S\,=\,K_1\left(NVU\right)^{1/3}$
- $ S\,=\,K_2\left(\frac{NU}{V}\right)^{2/3} $
- $ S\,=\,K_3\left(\frac{V^3}{NU}\right)$
- $ S\,=\,N{\rm{\ln}}\left(\frac{UV}{N^2K_4}\right)$
$K_i$'s are positive constants so that dimensions match. $S,U,N,V $ are the entropy, internal energy, number of particles and volume respectively. |

|
22-01-13 18:01:44 |
n |
|
|
[QUE/TH-13002] TH-PROBLEMNode id: 5173pagea) An ion of mass m and electric charge e is moving in a dilute gas of molecules with which it collides. The mean time between collisions is $\tau$. Let there be a uniform electric field $E$ along the x-axis. Show that the mean distance travelled by the ion is
$$ \frac{Ee}{m}\tau^2$$
assuming the velocity of the ion is zero immediately after collision. |

|
22-01-13 18:01:49 |
n |
|
|
[QUE/TH-13001] TH-PROBLEMNode id: 5172pageEstimate how long it would take a Nitrogen molecule of air in a room to travel 1 m?
Assume that the cross section of a molecule as $10^{-20}m$ and the temperature is $300^o$ K. |

|
22-01-13 18:01:24 |
n |
|
|
[QUE/TH-13003] TH-PROBLEMNode id: 5174pageDerive the fundamental relation
\[ S=\frac{S_0N}{N_0} + NR \ln[\Big(\frac{U}{U_0}\Big)^{3/2}\Big(\frac{V}{V_0}\Big)\Big(\frac{N}{N_0}\Big)^{-5/2} ] \]
for a perfect gas. |

|
22-01-13 17:01:01 |
n |
|
|
[QUE/TH-13004] TH-PROBLEMNode id: 5175pageThe fundamental equation for a system is given by
\begin{equation*}
u = \Lambda \frac{s^{3/2}}{v^{1/2}}
\end{equation*}
where \(\Lambda\) is a constant.
Prove the following equations
\begin{eqnarray}
T &=& \frac{5}{2} \frac{\Lambda S^{3/2}}{NV^{1/2}}\\
P V^{2/3} &=& N \frac{N \Lambda 2^{1/2}}{5*{3/2}} T^{5/3}\\
\mu &=& - \Big(\frac{2}{5}\Big)^{5/2} \frac{2}{\Lambda ^{2/3}} \Big(\frac{V}{N}\Big)^{1/3} T^{5/3}.
\end{eqnarray} |

|
22-01-13 17:01:57 |
n |
|
|
[QUE/TH-12001] TH-PROBLEMNode id: 5086pageConsider a $1m^3$ cubical box at STP. Estimate the number of molecules strike one wall in one second. Take $m$, the mass of the gas to be that of Nitrogen. |

|
22-01-13 17:01:59 |
n |
|
|
[QUE/TH-12002] TH-PROBLEMNode id: 5088pageA box is divided into two equal parts by a non-conducting partition having a hole of diameter $D$. Initially, some Helium gas is preset on both sides at temperature $T_1$ K and $T_2$K, by heating the walls of the box.
(a) What is the steady state when $D\,>>\,L_1$ and $D\,>>\,L_2$ where $L_1$ and $L_2$ are mean free paths at temperature $T_1$ and $T_2$ respectively.
(b) What is the steady state when $D\,<<\,L_1$ and $D\,<<\,L_2$ where $L_1$ and $L_2$ are mean free paths at temperature $T_1$ and $T_2$ respectively. |

|
22-01-13 17:01:49 |
n |
|
|
[QUE/TH-04001] TH-PROBLEMNode id: 5043pageConsider a closed cylinder whose walls are adiabatic. The cylinder is divided into three equal parts $A_1$, $A_2$ and $A_3$ by means of partitions $S_1$ and $S_2$, which can move along the length of the cylinder without friction. The partition $S_1$ is adiabatic and $S_2$ is conducting. Initially, each of the three parts contain one mole of Helium gas, which can be treated as an ideal gas, is at pressure $P_0$, temperature $T_0$ and volume $V_0$. Assume the specific heat at constant volume $C_v\,=\,\frac{3R}{2}$ and the specific heat at constant pressure $C_p\,=\,\frac{5R}{2}$. Now, heat is supplied to the to the left most partition $A_1$ till the temperature in part $A_3$ becomes $T_3\,=\,\frac{9T_0}{4}$.
(a) Find the final volume, pressure and temperature in terms of $V_0$, $P_0$ and $T_0$. Assume the entire process is quasistatic.
(b) What is work done by the gas in $A_1$ ?
(c) What is the heat supplied to the gas in $A_1$?
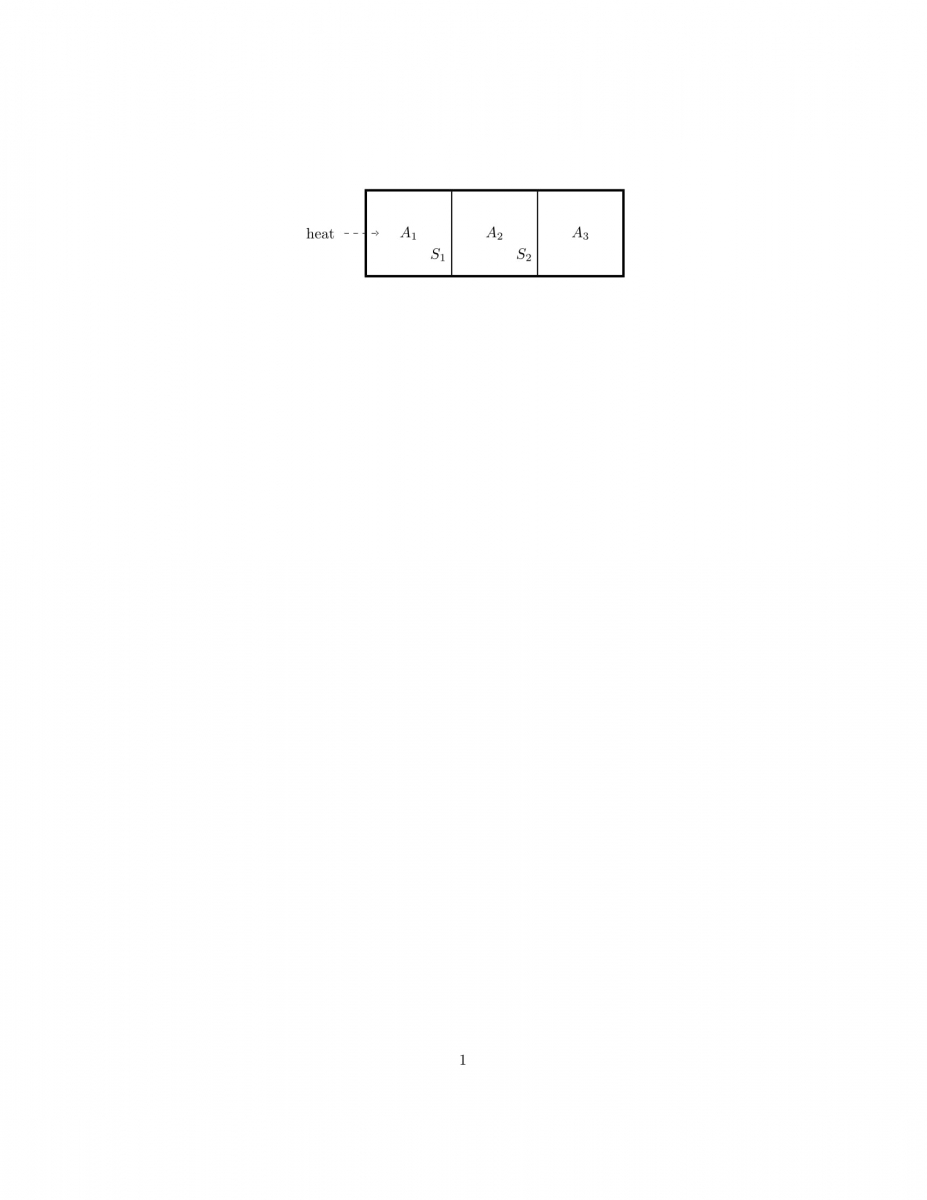 |

|
22-01-13 17:01:29 |
n |
|
|
[QUE/TH-01003] TH-PROBLEMNode id: 5153pageLet
$$\frac{\partial (x,y)}{\partial (a,b)}\,\equiv\,\left|\begin{array}{ll}
\frac{\partial x}{\partial a}&\frac{\partial y}{\partial a}\\
\frac{\partial x}{\partial b}&\frac{\partial y}{\partial b}\\
\end{array}\right|$$
Then show that
$$ \frac{\partial (x,y)}{\partial (a,b)}\frac{\partial (a,b)}{\partial (c,d)}\,=\,\frac{\partial (x,y)}{\partial (c,d)} $$
Remarks : 1. This can be generalised to higher dimensions.
2. This can be found in books - and is very useful in changing variables in multiple integrals. |

|
22-01-13 17:01:58 |
n |