QUE/TH
| Title | Name |
Date |
|---|---|---|
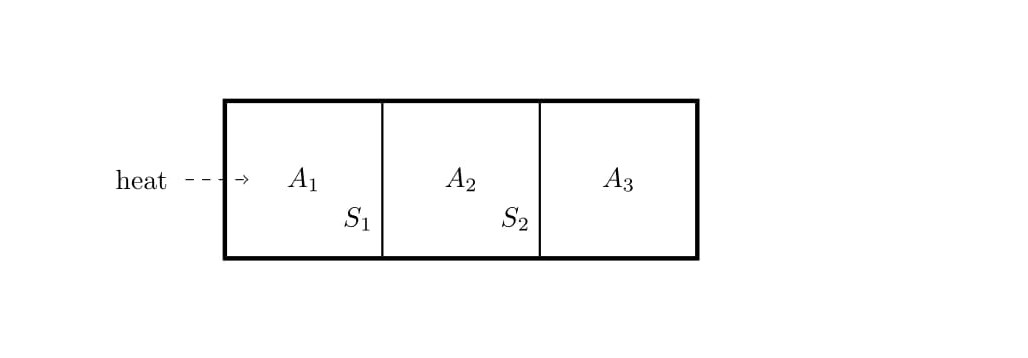
[QUE/TH-02001] TH-PROBLEMConsider a closed cylinder whose walls are adiabatic. The cylinder is divided into three equal parts $A_1$, $A_2$ and $A_3$ by means of partitions $S_1$ and $S_2$, which can move along the length of the cylinder without friction. The partition $S_1$ is adiabatic and $S_2$ is conducting. Initially, each of the three parts contain one mole of Helium gas, which can be treated as an ideal gas, is at pressure $P_0$, temperature $T_0$ and volume $V_0$. Assume the specific heat at constant volume $C_v\,=\,\frac{3R}{2}$ and the specific heat at constant pressure $C_p\,=\,\frac{5R}{2}$. Now, heat is supplied to the to the left most partition $A_1$ till the temperature in part $A_3$ becomes $T_3\,=\,\frac{9T_0}{4}$ Find the final volume, pressure and temperature in terms of $V_0$, $P_0$ and $T_0$. Assume the entire process is quasistatic. (a) What is work done by the gas in $A_1$ ? (b) What is the heat supplied to the gas in $A_1$? |

|
22-01-14 10:01:44 |
[QUE/TH-05003] TH-PROBLEMConsider $n$ mole of ideal gas whose entropy is given by $$ \frac{n}{2}\left[c_1\,+\,5R{\ln}\frac{U}{n}\,+\,2R{\ln}\frac{V}{n} \right]$$ where $R$ is the univeral gas constant, $U$ the internal energy, $V$ the volume and $c_1$ a constant.
|

|
22-01-14 10:01:59 |






 ||Message]
||Message]