[NOTES/QM-16006] Energy Levels in Spherically Symmetric Potentials Accidental Degeneracy

                              Contents

1. General Properties of Bound State Spectra
2. Bound state spectrum
3. Coulomb problem spectrum
4. Accidental degeneracy 

1. General Properties of Bound State Spectra. 

A potential is spherically symmetric if in polar variables it depends only on $r$ and not on $\theta$ and $\phi$ coordinates . We shall now discuss general properties of solution of 3-dimensional Schr\"{o}dinger equation $ H\psi = E\psi $ where

$$H = {\vec{p}\,^2\over 2m} + V(r) $$
and the potential $V(r)$ is spherically symmetric.

Conserved quantities 

We note that all the three components of $\vec{L}$ commute with Hamiltonian $$ {} [\vec{L} , H] = 0 $$ hence $$ {} [\vec{L}^2 , H] = 0\,\,\,. $$ The parity operator $\mathscr{P} $ $$ \mathscr{P} \psi(\vec{r}) = \psi(-\vec{r}) $$ also commutes with $L^2L_z$ and $H$, operators. Therefore, the eigen functions of $H$ will also be eigen functions of $L^2, L_z$ and parity and each level can be assigned a definite value of $l, m$ and parity. For a state with definite value of $l$, the value of parity is $\equiv(-1)^l$. In this case $L^2, L_z$ and $H$ form a complete commuting set. {$(2\ell+1)$ degeneracy } We use the notation $\ket{El,m}$ to denote the simultaneous eigenvector of $H, L^2$ and $L_z$ \begin{eqnarray} H\ket{E,l m} & = & E\ket{E,l m}\\ L^2\ket{E,l m} &=& l(l+1)\hbar^2\ket{E,l m}\\ \mbox{and}~~ L_z\ket{E,l m} &=& m\hbar\ket{E,l m}\\ \mathscr{P} \ket{E,l m} &=& (-1)^l\ket{E,l m} \end{eqnarray} Applying $L_-$ on $\ket{El,m}$ several times leads successively to \begin{eqnarray} \ket{E l, m-1>}~,~\ket{E,l,m-2} &\cdots& |E l, -l\rangle\\ \end{eqnarray} and the action of $L_+$ on $\ket{E,l m}$ leads to the states \begin{eqnarray} \ket{E l, m+1}~,~\ket{E,l,m+2} &\cdots& |E l, l\rangle \end{eqnarray}
All these states will have the same value of energy. This statement can be proved by making use of the fact that $H$ commutes with $L_\pm$ and that action of $L_+$ (or $L_-$) on $|Elm>$ leads to states $|El,m+1>$ (or $|El,m-1>$). Thus we see that the bound state energy eigenvalues of a spherically symmetric potential problem with have $(2l+1)$ fold degeneracy. ( What about the continuous energy eigenvalues? )

Radial wave function

The Schr\"odinger equation for a spherically symmetric potential can be solved by separation of variables in polar coordinates. The angular part of the wave functions turns out to be a spherical harmonic $Y_{lm}(\theta,\phi)$ and the wave function has the form $$ \psi(r,\theta,\phi) = R(r)Y_{lm}(\theta, \phi) $$ where $R(r)$ is radial wave function satisfying the Schr\"odinger equation $$ -{\hbar^2\over2m} {1\over r^2} {d\over dr} r^2{d\over dr} R(r) + \left(V(r) + {l(l+1)\hbar^2\over2mr^2}\right) R(r) = ER(r)\,\,\,. $$ If we define $\chi(r)=rR(r)$, the function $\chi$ satisfies the equation $$-{\hbar^2\over2m} {d^2\chi\over dr^2} + \left(V(r) + {l(l+1)\hbar^2\over2mr^2}-E\right)\chi = 0\,\,\,.$$ with boundary condition $\chi(r)|_{r=o}=0$, otherwise $R(r)$, the radial wave function will tend to $\infty$ as $r\to\infty$.

2. Bound state spectrum.
The equation for $\chi$ has the form of one dimensional Schr\"odinger equation. Let $n^{'}$ denote the number of zeros of the radial wave function, excluding $r=0$ and at $r=\infty$. Then for a fixed value of $l$, the energy will increase with $n^{'}$, $n^{'}=0,1,2,\cdots$ will correspond to, for a fixed $l$, the `ground' state, first excited state, the second excited state etc. Because of the $l$ dependence of the term ${l(l+1)\hbar^2\over2mr^2}$ in the potential appearing in equation for $\chi(r)$, we expect that as $l$ is changed, keeping the number of nodes to be the same, $E$ would also change. Increasing $l$ would lead to increase in $E$, when $n^{'}$ is kept fixed.

Thus the spectrum would appear as follows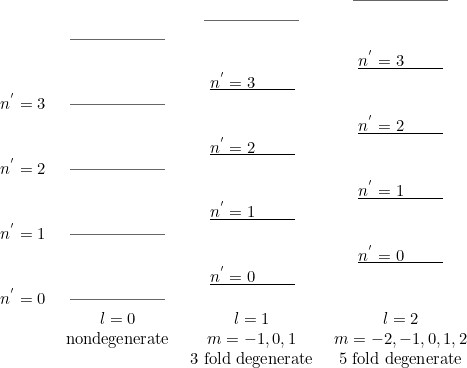

3. Coulomb problem spectrum

For hydrogen atom the energy levels are given by $$ E = -{Z^2
e^4m\over2\hbar^2(n^{'}+l+1)^2}$$ The energy does not depend on $n^{'}$
and $l$ separately but only on the combination $n=(n^{'}+l+1)$. For a fixed
$n$, $l$ can have values $0,1,\cdots,n-1$ ( because $n^{'}\ge0$ ) and all
these solutions correspond to the same energy eigenvalue. The energy level
diagram of H-atom, therefore, appears as shown below.

Putting all the levels which have the same energy together we get the following schematic representation of energy levels of $H$ atom. This table also shows that the allowed values of $l$ for each $n$, and number of $m$ values for each level. The number of total $m$ values, with the same energy, is $n^2$ and the degeneracy, after taking spin into account, becomes $2n^2$.



4. Accidental degeneracy.

Comparing the hydrogen atom levels with those of a general spherically symmetric potential, we find that energies for states with several different values of $l~ (=0,1,2 \dots n-1)$ are the same. For a general spherically symmetric potential different combinations of $n,l$ values correspond to different bound state energies, and are $(2l+1)$ fold degenerate. Thus there is an extra degeneracy is present for H atom beyond the expected $(2l+1)$ fold degeneracy this phenomenon present in the case of hydrogen atom is known as {\it accidental degeneracy.} Another well known case of accidental degeneracy is that of isotropic harmonic oscillator ( $ V(r)= {1\over 2} kr^2$) in three dimensions.

 Remarks

1. It must be emphasized that the accidental degeneracy is due to the special symmetry of the Coulomb problem.
2. Any slight deviation of the potential from ${1\over r}$ will result in splitting of energy levels with different values of $l$.
3. It is known that the accidental degeneracy is present whenever the Schr\"odinger equation $H\psi=E\psi$ can be separated into ordinary differential equations in more than one set of coordinate system. \noindent $H$ atom --- Separation of variables for the Coulomb problem is possible in
   1. spherical polar coordinates $r,\theta,\phi$
   2. parabolic coordinates $\xi, \eta, \phi$ defined by \begin{eqnarray} \xi = r-z = r(1-\cos\theta)\quad \eta = r+z =r(1+\cos\theta)\quad \phi = \phi \end{eqnarray}
4. The isotropic oscillator also exhibits accidental degeneracy. For isotropic harmonic oscillator,$V(r) ={1\over 2} k r^2$, the Schrodinger equation can be separated in two set of variables, Cartesian and spherical polar coordinates.