Category:
Examples of application of Noether's theorem are given for mechanical systems. The following relationship between symmetry and corresponding conservation law is demonstrated by means of explicit examples of system consisting of finite number of particles.
$\newcommand{\HighLight}[2][]{\text{[#2]}}$
For a system of $N$ particles interacting via potential $V({|\vec x_\alpha}-\vec{x_\beta}|)$ will find symmetries and corresponding conservation laws. \begin{equation}\label{EQ01} L(x,\dot{x})=\frac{1}{2}\sum_\alpha m_\alpha\dot{\vec{x}}_\alpha^{\,2} -\sum_{\alpha<\beta}V(|\vec{x_\alpha}-\vec{x_\beta}|) \end{equation}
Translation symmetry
Consider the translation transformations \begin{equation}\label{EQ02} \vec{x}_\alpha\rightarrow \vec{x}_\alpha^{\,\prime}=\vec{x}_\alpha-\vec{a}. \end{equation} Then we have \begin{equation} \dot{\vec{x}}_\alpha^{\,\prime}=\dot{\vec{x}}_\alpha, \qquad |\vec{x}_\alpha^{\,\prime}- \vec{x}_\beta^{\,\prime}| = |\vec{x}_\alpha-\vec{x}_\beta|. \end{equation}
Translations are a symmetry transformations of the Lagrangian \eqref{EQ01}. To see this consider
\begin{eqnarray}
L(\vec {x}^{\,\prime},\dot{\vec{x}}^{\,\prime})
&=&\frac{1}{2}\sum_\alpha m_\alpha\dot{\vec{x}}_\alpha^{\,\prime2}-
\sum_{\alpha,\beta}
V(|\vec{x}_\alpha^{\,\prime}-\vec{x}_\beta^{\,\prime}|)\\
&=&\frac{1}{2}\sum_\alpha m_\alpha\dot{\vec{x}}_\alpha^{\,\prime2}-
\sum_{\alpha,\beta}V(|\vec {x}_\alpha-\vec{x}_\beta^{\,\prime}|)\\
&=&L(\vec x,\dot{\vec{x}}).
\end{eqnarray}
Therefore, the Lagrangian invariant is invariant under translations.
The constant of motion corresponding to space translations is easily found. The conserved quantities are
\begin{eqnarray}
\sum \frac{\partial{L}}{\partial{\dot q_\alpha}}\delta q_\alpha=\sum
m_\alpha\dot{\vec{x_\alpha}}.\vec a
=\vec a.\sum m_\alpha\dot{\vec{x_\alpha}}
\end{eqnarray}
\begin{equation} \frac{d}{dt}\vec{a}.\vec{p}=0 \Rightarrow \vec{a}.\frac{d}{dt}\vec{p}=0. \end{equation} Since $\vec{a}$ is arbitrary and independent we get the result that conserved quantity corresponding to translations is \begin{equation}\label{EQ09} \frac{d}{dt}\vec p=0.\qquad\qquad \mbox{\text{Prove This.}} \end{equation} where $\vec{p}=\sum_km_\alpha \dot{\vec{x}}_\alpha$ is the total momentum.
Thus we see that invariance under translations gives rise to conservation of total momentum.
For later use, we note that the total momentum is same as \(M\dot {\vec{X}} \), where \(M\) is the total mass and \(\vec{X}\) is the position of the centre of mass. Conservation of total momentum implies that the velocity \(\dot{\vec{X}}\) is constant.
Rotations
Let a set of axes $K^{\,\prime}$ be obtained from a set $K$ by applying rotation about $X_3$ axis by an angle $\theta$ then
\begin{eqnarray}
x^{\,\prime}_{\alpha 1} &=&x_{\alpha 1}\cos\theta + x_{\alpha 2}\sin\theta\\
x^{\,\prime}_{\alpha 2} &=& -x_{\alpha 1}\sin\theta + x_{\alpha 2}\cos\theta\\
x^{\,\prime}_{\alpha 3} &=&x_{\alpha 3}\end{eqnarray}
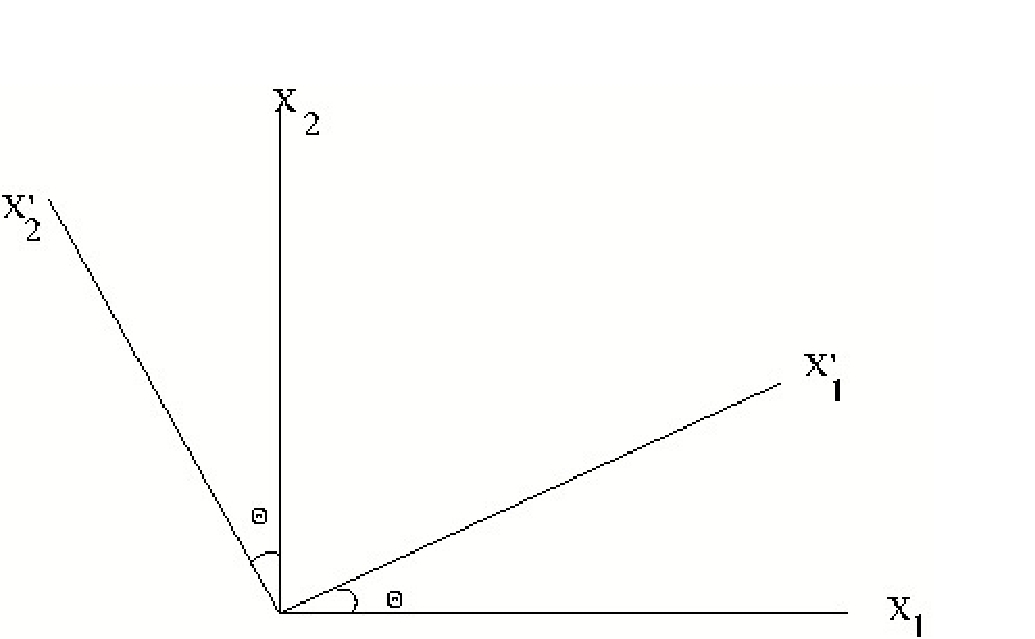
Note that
\begin{eqnarray}
{\dot{x}_{{\alpha 1}}^{\,\prime2}}+{\dot{x}_{{\alpha
2}}^{\,\prime2}} + x_{\alpha3}^{\prime2}
&=&(\dot{x}_{\alpha 1}\cos\theta+\dot{x}_{\alpha 2} \sin
\theta)^2+(-\dot{x}_{\alpha 1}\sin\theta+\dot{x}_{\alpha 2} \cos
\theta)^2+x_{\alpha3}^{\,2}\\
&=&\dot{x}_{{\alpha 1}}^{\,2}+\dot{x}_{{\alpha2}}^{\,2} +
\dot{x}_{\alpha3}^{\,2}
\end{eqnarray}
Similarly, \begin{equation} {|\vec x_\alpha}^{\,\prime}-\vec{x_\beta}^{\,\prime}|^2={|\vec x_\alpha}-\vec{x_\beta}|^2 \qquad {\dot{\vec{x}}_\alpha^{\,\prime2}}=\vec{x}_\alpha ^2\\ \end{equation}
This implies \begin{equation} L(x^{\,\prime}_\alpha,\dot {x}^{\,\prime}_\alpha)=\frac{1}{2}\sum m_\alpha\dot{\vec{x}}_\alpha^{\,2}+V(|{x_\alpha}-{x_\beta}|)= L(x_\alpha,\dot {x}_\alpha) \end{equation} Therefore, the Lagrangian is invariant under rotations. Let us now compute the variations for small \(\theta\):
\begin{eqnarray}
\delta x^{\,\prime}_{\alpha 1}&=&x_{\alpha 1}\cos\theta+x_{\alpha 2}\sin
\theta-x_{\alpha 1}\\
&\cong& \theta x_{\alpha 2}+O({\theta}^2)\\
\delta x^{\,\prime}_{\alpha 2}&=&-x_{\alpha 1}\sin\theta+x_{\alpha 2}
\cos\theta-x_{\alpha
2}\\
&\cong& -\theta x_{\alpha 1} + O({\theta}^2);\\
\delta x_{\alpha 3}&=&0\\
\end{eqnarray}
Therefore, conserved quantity is
\begin{eqnarray}
G&=&\sum_{\alpha ,k}\frac{\partial{L}}{\partial{\dot {x}_{\alpha,k}}}\delta
x_{\alpha k} \\
&=&\sum_\alpha \Big(\frac{\partial{L}}{\partial{\dot {x}_{\alpha 1}}}\delta
x_{\alpha 1}+\frac{\partial{L}}{\partial{\dot {x}_{\alpha 2}}}\delta x_{\alpha
2}+\frac{\partial{L}}{\partial{\dot {x}_{\alpha 3}}}\delta x_{\alpha 3}\Big)\\
&=&\theta \sum_\alpha\Big(m _\alpha\dot {x}_{\alpha 1}x_{\alpha 2}-m _\alpha\dot
x_{\alpha 2}x_{\alpha 1}\Big)\\
&=&\theta \sum_\alpha m _\alpha\Big(\dot {x}_{\alpha 1}x_{\alpha 2}-\dot
x_{\alpha 2}x_{\alpha 1}\Big)\\
&=&\theta \sum_\alpha\Big(\dot p_{\alpha 1}x_{\alpha 2}-\dot p_{\alpha
2}x_{\alpha 1}\Big) \\
&=&\theta\sum_\alpha\Big(\vec x_\alpha \times \vec p_\alpha \Big)_3
\end{eqnarray}
\begin{equation} \frac{d G}{dt}=0 \Rightarrow \frac{d}{dt}\sum_\alpha\Big(\vec x_\alpha \times \vec p_\alpha \Big)_3=0 \end{equation} Therefore, the third component of angular momentum $L_3=\sum_\alpha\Big(\vec x_\alpha \times \vec p_\alpha \Big)_3 $ is a constant of motion.
Similarly, invariance under rotations about other axes leads to conservation of other components of angular momentum.
Galilean Transformation
The Galilean transformation from a frame to another frame moving with velocity \(\vec{v}\) is given by a frame $$\vec{x}^{\,\prime}=\vec{x}-\vec{v}t$$ where $\vec{v}$ is independent of time. Therefore, $$\dot {\vec x}^{\,\prime}=\dot{\vec{x}}-\vec v$$ and $$ \delta \vec x ^{\,\prime}= -vt;\qquad\delta \dot {\vec x}^{\,\prime}=-v. $$ Since $V(|\vec x_\alpha^{\,\prime}-\vec x_\beta^{\,\prime}|) =V(|\vec x_\alpha-\vec x_\beta|)$, the change in Lagrangian is given by
\begin{eqnarray}
\delta L &=& L(\vec{x}^{\,\prime},\dot{\vec{x}}^{\,\prime})-L(\vec{x},
\dot{\vec{x}})\\
&=&\frac{1}{2}\sum m_\alpha\dot{\vec{x}}_\alpha^{\,\prime\,2}
- \frac{1}{2}\sum m_\alpha\dot{\vec{x}}_\alpha^{\,2}\\
&=&
\frac{1}{2}\sum_\alpha\Big(m_\alpha(\dot{\vec{x}}_\alpha -\vec{v})^2 -
\frac{1}{2}m{\dot{\vec{x_\alpha}}\,^{2}}\Big)\\
&=&-\sum_\alpha (m_\alpha\dot{\vec{x}}_\alpha)\cdot \vec v + O(v^2).
\end{eqnarray}
Thus \begin{equation} L(\vec{x}^{\,\prime},\dot{\vec{x}}^{\,\prime}) = L(\vec x_\alpha,\dot{\vec{ x_\alpha}})-\underbrace{\frac{d}{dt}\sum m_\alpha \vec x_\alpha.\vec v}_{\frac{d}{dt}\Omega} \end{equation} and we have identified \(\Omega\) as indicated above. Therefore, conserved quantity is
\begin{eqnarray}
G&=&\sum_{\alpha, k} \frac{\partial{L}}{\partial{\dot{x}_{\alpha k}}}\delta x_{\alpha k}-\Omega \\
&=&-\sum_{\alpha, k} \dot{x}_{\alpha k} (v_k t) +\sum m_\alpha \vec{x}_\alpha.\vec{v}\\
&=&-\vec{v}\Big(\sum m_\alpha\dot{\vec{x}}_{\alpha}\Big) t-\sum m_\alpha
\vec{x}_\alpha
\end{eqnarray}
Therefore, the quantity $$\vec v\cdot\Big(\sum m_\alpha\dot{\vec x}_{\alpha }\Big) t-\sum m_\alpha \vec x_\alpha$$\\ is independent of time.
To understand the above result a little, let us recall that the definition of the coordinate of centre of mass implies \begin{equation} \sum_\alpha m_\alpha \vec{x}_\alpha= M \vec{X} \qquad \text{and} \quad \sum_\alpha m_\alpha \dot{\vec{x}}_\alpha= M \dot{\vec{X}}. \end{equation} where \(M\) is the total mass of the system. Thus we have $$\vec{v}\cdot\big (\dot{\vec X } t-\vec X\big)= \text{constant}.$$ Since \(\vec{v}\) is arbitrary, we get \begin{equation} \vec{X}= \dot{\vec{X}}t+\text{constant}, \qquad \qquad \HighLight{Write a proof!} \end{equation} Note that at the end of Sec.1, we have already seen that the velocity \(\dot {\vec{X}}\) is constant in time. Therefore $\vec{X}$ is a linear function of time. {\it Thus the conservation law associated with invariance of Galilean transformations is that the centre of mass of the system moves with a constant velocity $\dot{\vec X}.$}






 ||Message]
||Message]
