[NOTES/EM-04013] Example --- The Method of Images

The figure  below shows a thick shell, with radii \(a<b\), held at zero potential. A point charge \(Q\) is placed at the centre of the shell. The problem is to find the potential everywhere inside the shell, i.e.  for \(r< a\). In this case the entire region \(a \le r\le b\) will  be at zero potential. No set of image charges can be found such that the entire conductor has zero potential due to  the charge \(Q\) and the image charges. However, this is not required to find the solution by the method of images.

To solve the problem, it should be noted that the boundary of region of interest \( 0< r < a\) is the inner surface of the shell. By uniqueness theorem it is sufficient to find image charges such that the potential on the inner surface remains zero under the combined effect of the point charge \(Q\) and image charges.

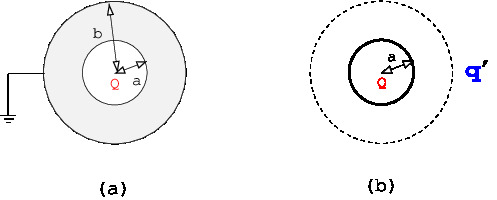
The rest of the solution follows as in the case of point charge outside a conducting sphere held at zero potential.