[NOTES/EM-04012] Pressure on A Charged Conducting Surface

Given a set of point charges \(q_k\), the force let \(\vec E_k\) denote the electric field due to the charge \(q_k\). The force \(F_m\), on a particular charge \(q_m\), is given by \(q_m\vec E^\prime\) where \(\vec E^\prime\) is the field due to all charges except that due to the charge \(q_m\) itself. \begin{equation} F_m = q_m \sum_{k\ne m} E_k=q_m\vec E^\prime \end{equation}

The sum \(\sum_{k\ne m} E_k\), being the field due to all charges other that \(q_m\), can obviously written as \begin{equation} \sum_{k\ne m} E_k = \sum_{ \text{all\,} k} E_k - E_m \end{equation}

Suppose \(S\) is a closed conducting surface carrying a charge density \(\sigma\), in general, varying from point to point. Given a point \(P\), we wish to compute the Coulomb force per unit area, due to the charge on the entire surface \(S\), at a given point \(P\). Since charge is continuously distributed, the required computation has to be done with some care.

Consider a small area \(\Delta S\), a circle of radius \(\epsilon_1\), at a point \(P\). The charge on this surface \(\Delta S\) will feel a force due to the charge on rest of the surface.

As explained for the point charges, the force on \(\Delta S\) must be computed by taking into account of charge on the surface excluding the element \(\Delta S\) itself. Thus the Coulomb force on \(\Delta S\) is given by the  charge on \(\Delta S\) \(\times\) the electric field due to the rest of the surface.

As for the case of point charges discussed in the beginning, the electric field due to the rest of the surface will be called \(E_{S^\prime}\), and is given by \begin{equation} \vec E_{S}^\prime = \vec E_\text{Full S} - \vec E_{\text{Charge in } \Delta S}. \end{equation}

The field due to the full surface, the first term, is normal to the surface and has magnitude \(\sigma/\epsilon_0\), as shown in Fig(b).

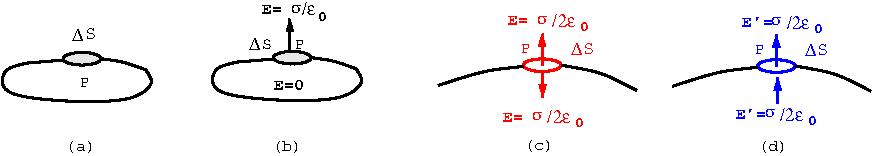
Now to compute the second term, i.e. the field due to the charge on \(\Delta S\) we will find the field at a point \(P\), a distance \(\epsilon_2\) from this surface. It is understood that at the end, the limit \(\epsilon_1, \epsilon_2 \to 0\) is taken. From a small distance \(\epsilon_2\), the surface appears like an infinite plane sheet, (single layer) of charge density \(\sigma\). Thus the field at the nearby point \(P\) is, therefore, \(\sigma/(2\epsilon_0)\). as shown in Fig.(c).

The field \(\vec E^\prime\) at points near \(P\), is therefore the difference of field in (b) and (c), is given by \(\sigma_0/2\epsilon_0\). This electric field is seen to be continuous, as shown in Fig(d). Therefore, in the limit \(\epsilon_1, \epsilon_2 \to 0\), the electric field for computing the force on \(\Delta S\) is seen to be \(\sigma/(2\epsilon_0)\) as shown in Fig(d)

The force on the charge in surface element, \(\Delta S\) at a point \(P\) is, therefore given by, \((\sigma \Delta S) \vec E^\prime\). Hence \begin{equation} \text{Force per unit area}= \frac{\sigma^2}{2\epsilon_0}. \end{equation}