Reading Assignment :
Sections 4.1 to 4.3 of Callan
7. Consider $n$ mole of ideal gas whose entropy is given by $$ \frac{n}{2}\left[c_1\,+\,5R{\ln}\frac{U}{n}\,+\,2R{\ln}\frac{V}{n} \right]$$ where $R$ is the univeral gas constant, $U$ the internal energy, $V$ the volume and $c_1$ a constant.
(a) Calculate the specific heats $c_V$ and $c_p$
(b) A room is at a temperature $273^o$ K which is in equilbrium with the surroundings. One hour after turning on a heater, the room is at $300^o$ K. Assuming the air is described by the equation given above, find the energy density for the two different temperatures. Answer should be in terms of the atmospheric pressure $P_0$, assume it is constant and the room is always at $P_0$.
8. Two heat reservoirs, each of fixed volume $V$ are at temperatures $T_1$ and $T_2$ ( $T_1\,>\,T_2$) initially. An engine operates between the two till both of them reach the same temperature $T_3$. The specific heat $c_V$ of the reservoirs can be assumed to constant through out. The engine is back to its inital state. Show that $$ T_3^2\,\geq\,T_1T_2 $$
9. ( Refer to the problem 8) Calculate the maximum work that can be extracted from the two reservoirs. ( Answer in terms of $T_1$ and $T_2$)
(a) $$S\,=\,K_1\left(NVU\right)^{1/3}$$
(b) $$ S\,=\,K_2\left(\frac{NU}{V}\right)^{2/3} $$
(c) $$ S\,=\,K_3\frac{V^3}{NU}$$
(d) $$ S\,=\,N{\rm{\ln}}\left(\frac{UV}{N^2K_4}\right)$$
$K_i$'s are positive constants so that dimensions match. $S,U,N,V $ are the entropy, internal energy, number of particles and volume respectively.
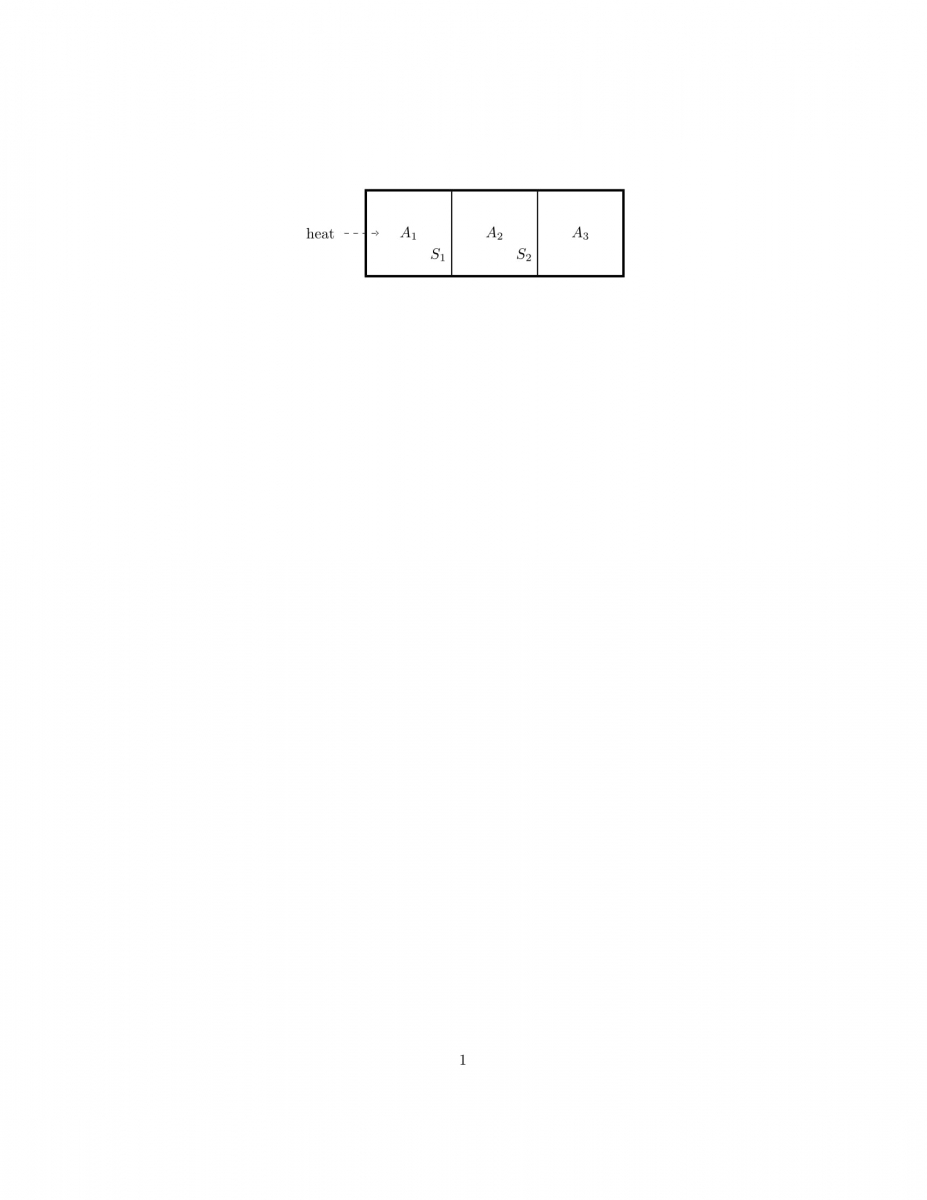
5. Consider a closed cylinder whose walls are adiabatic. The cylinder is divided into three equal parts $A_1$, $A_2$ and $A_3$ by means of partitions $S_1$ and $S_2$, which can move along the length of the cylinder without friction. The partition $S_1$ is adiabatic and $S_2$ is conducting. Initially, each of the three parts contain one mole of Helium gas, which can be treated as an ideal gas, is at pressure $P_0$, temperature $T_0$ and volume $V_0$. Assume the specific heat at constant volume $C_v\,=\,\frac{3R}{2}$ and the specific heat at constant pressure $C_p\,=\,\frac{5R}{2}$. Now, heat is supplied to the to the left most partition $A_1$ till the temperature in part $A_3$ becomes $T_3\,=\,\frac{9T_0}{4}$ Find the final volume, pressure and temperature in terms of $V_0$, $P_0$ and $T_0$. Assume the entire process is quasistatic.
3. ( Continuation of problem 2)
(a) What is work done by the gas in $A_1$ ?
(b) What is the heat supplied to the gas in $A_1$?






 ||Message]
||Message]
