An Introduction to Dirac Delta Function

The standard definition of a function $f$ is as a mapping from the set ${\mathcal R}$ of real numbers (or a suitable interval of it) into the real numbers, so that it assigns a number $f(x)$, called its value, to $x\in{\mathcal R}$.

\begin{eqnarray}\begin{array}{ll}\theta(x)=0&x<0\\\theta(x)=1&x>0\end{array}\end{eqnarray}
This is very much like an ordinary function, in fact a constant function, everywhere except the point $x=0$ where it is not defined. The function is discontinuous at $x=0$ and  we can not define its derivative at that point.

The theory of generalised functions is a genralization of the concept of functions to include functions which may have discontinuities or singularities at some or other point of their domain of definition.

For this purpose we must look at an alternative way to define a function. There are three different ways to define generalized functions.

1. A generalized function is defined by a sequence of ordinary functions which "tend towards'' the singular function
2. A generalized function defined indirectly when integral of its product with a smooth well behaved functions is given.
3. A generalized function is defined as  boundary value of an analytic function.

 All three methods are used and they complement each other.Sequence of functions.The best example is the Dirac delta function. The sequence of functions is chosen as

\begin{eqnarray}f_n(x)=\sqrt{\frac{n}{\pi}}e^{-nx^2},\qquad n=1,2,\dots \end{eqnarray}

The functions looks like



The "area under the curve'' of $f_n$ is $\int_{-\infty}^\infty f_n(x)dx=1$  (check that). And for larger values of $n$ the functions become narrowly and sharply peaked around $x=0$ always keeping the area under the curve equal to 1. The Dirac delta function is the limiting function of this sequence.

This is not the only sequence of functions which defines the Dirac delta. There are several (in fact infinitely many) such sequences.  Another sequence of functions is obtained by
\begin{eqnarray}\frac{1}{\pi}\frac{\epsilon}{x^2+\epsilon^2} \end{eqnarray} as $\epsilon\to 0$. If you really must insist on a sequence, you can take $\epsilon=1/n$
which is equivalent to $\epsilon\to 0$ as $n\to \infty$. This gives\begin{eqnarray}g_n(x)= \frac{n}{\pi}\frac{1}{1+n^2x^2},\qquad n=1,2,\dots \end{eqnarray} 
These functions also have unit area under the curve and for large values of $n$ the functions become very sharply peaked and narrow near $x=0$.

 Actually it does not matter which particular sequence is used or the definition. 

The step function can be approximated by a sequence of functions
\begin{eqnarray}h_n(x)=\frac{1}{2}+\frac{1}{2}\tanh(nx) \end{eqnarray}
The function $\tanh(nx)$ looks like

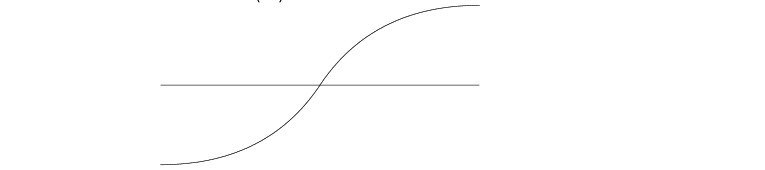
For large values of $n$ the function becomes more and more steep at origin and for most of the positive side it is practically equal to $1$ and on
the negative side it is $-1$. Another sequence is
\begin{eqnarray*}k_n(x)=\frac{1}{2}+\frac{1}{\pi}\tan^{-1}(nx) \qquad n=1,2,\dots\end{eqnarray*}

where it is understood that we take the values of $\tan^{-1}(x)$ in the range $-\pi/2$ to $\pi/2$. The function  $\tan^{-1}(x)$ also has  graph   like $\tanh(x)$, and suitable normalization has been done to secure the right values in the limit to give the step function.

A third interesting example is the "Cauchy principal value'' of $1/x$, This function is obtained by omitting the singular part of $1/x$ in a symmetrical way from the neighbourhood of $x=0$. Let $\epsilon$ be a small number, then we define the Caucy principal value denoted by 
\begin{eqnarray}P\left(\frac{1}{x}\right)\end{eqnarray} as the limit $\epsilon\to 0$ of the function

\begin{eqnarray*}P\left(\frac{1}{x}\right) &=& \frac{1}{x}\qquad (|x|>\epsilon)\\ &=& 0\qquad (|x|<\epsilon) \end{eqnarray*}

Again we see the discontinuities; We can define it by a sequence of functions.

\begin{eqnarray*}C_n(x)=\frac{x}{x^2+\epsilon^2}=\frac{n^2x}{1+n^2x^2}\qquad (\epsilon=\frac{1}{n}) \end{eqnarray*}

You must plot these functions. The idea is that for $|x|>>\epsilon$ the function behaves like $1/x$ and near $x=0$ it is linear with a large slope ($n^2$). The turning point from $1/x$ to $x$ behavior  is at $x=\epsilon=1/n$.

We are already in a position to prove an important relation :
\begin{eqnarray}\lim_{\epsilon\to 0}\frac{1}{x\pm
i\epsilon}=P\left(\frac{1}{x}\right) \mp
i\pi\delta(x) \end{eqnarray}
The left hand side is a a complex function with a small imaginary part 
\begin{eqnarray}\frac{1}{x\pm i\epsilon}=\frac{x}{x^2+\epsilon^2} \mp
i\frac{\epsilon}{x^2+\epsilon^2}\end{eqnarray}
When $\epsilon\to 0$ the first term on the right hand side becomes the Cauchy principal value, and the second term gives Dirac delta function. Therefore (as $\epsilon\to 0$)
\begin{eqnarray}\frac{1}{x\pm i\epsilon}=P\left(\frac{1}{x}\right)\mp
i\pi\delta(x) \end{eqnarray} 

There is another interesting fact we can derive. If a sequence $f_n$ of functions tends to a generalized function $f$ then we say that the sequence $f'_n$ of derivatives of the functions defines the {\bf derivative} $f'$ of the generalized function. You can check in this way that the derivative of the step function is the Dirac delta function :
\begin{eqnarray}\theta'(x)=\delta(x) \end{eqnarray}

The method of indirect definition of a generalized function is somewhat likethe way police obtains information on hard criminals through its informers who
are themselves  better behaved  but happen to be in the company of the those wanted men.

In this method the effect of the generalized function is seen when "it is smeared with a test function''. This means we integrate the generalized
function to be defined with a known well behaved "test'' function $\phi$ and give the value of the integral
\begin{eqnarray}(f,\phi)\equiv \int_{-\infty}^{+\infty} f(x)\phi(x) dx
\end{eqnarray}
The class ${\mathcal D}$ of test functions should be sufficiently large so that a knowledge of $(f,\phi)$ for all $\phi\in{\mathcal D}$ is enough to extract all knowledge of the generalized function $f$.

The class of functions ${\mathcal D}$ is taken to be the set of all functions which are infinitely differentiable and which vanish outside a finite interval. It is hoped that for functions with a singularity, the process of integrating with a very well behaved and smooth function $\phi$ will give meaningful result $(f,\phi)$, even though it may not be possible to define the function at all points by the usual definition.

For example, for our step function, the definition as a generalized function is 
\begin{eqnarray}(\theta,\phi)= \int_0^{+\infty} \phi(x) dx \end{eqnarray}
which is obvious in this simple case.

What is not obvious is that this definition can be used to define a derivative of the step function which is another generalized function. If there was a normal function $f(x)$ we would have written for its derivative
$f'=df/dx$
\begin{eqnarray}(f',\phi)=\int_{-\infty}^{+\infty} f'(x)\phi(x)
dx=[f\phi]_{-\infty}^{+\infty}-
\int_{-\infty}^{+\infty} f(x)\phi'(x) dx=-(f,\phi')\end{eqnarray}
the first term being zero because every $\phi$ vanishes at $\pm\infty$.  We use the above relation to define the derivative $f'$ of a generalised function $f$,
\begin{eqnarray}(f',\phi)=-(f,\phi')\end{eqnarray}
As an example we can define the derivative of the step function $\theta$ as 
\begin{eqnarray}(\theta',\phi)=-(\theta,\phi')=\int_0^{+\infty} \phi'(x)
dx=-[\phi(x)]_0^{\infty}
=\phi(0)\end{eqnarray}
Therefore $\theta'$ is a new generalized function, traditionally denoted by $\delta$ and called {\bf Dirac delta function}, (which Dirac had defined in 1930 to
replace the Kronecker delta $\delta_{ij}$ in quantum mechanics for the continuous case $\delta(x-y)$).
\begin{eqnarray}(\delta,\phi)=\phi(0)\end{eqnarray}
If we try to find the values of $\theta'(x)=\delta(x)$, it is zero for every $x<0$ or $x>0$. This is obvious because in these places the function $\theta$ is a
constant. The new thing about the definition of generalized function is the derivative  (a generalized derivative). We expect the derivative to go to infinity, because of the finite jump in the step function at $x=0$. The usual way to define delta function is to say that it is a function which is zero everywhere except at $x=0$ where it is infinity in such a way that the integral of the function ("area under the curve'') is unity
\begin{eqnarray}\int \delta(x) dx=1\end{eqnarray}
What is more $\delta$, being a generalized function itself, has its own derivative defined
\begin{eqnarray}(\delta',\phi)=-(\delta,\phi')=-\phi'(0) \end{eqnarray}
Thus we see that generalized functions have derivatives of all orders defined which is good progress considering that it was not possible to differentiate them even once by by the usual definition.

We shall prove a very important formula, which can be written as
\begin{equation}\delta(x)=\frac{1}{2\pi}\int_{-\infty}^{+\infty}\,e^{ikx}\,dk
\end{equation}
As things stand in this formula the integral on the right hand side is not well defined. This formula is very useful but symbolic. One way is to define it as the limit of convergent integrals 
\begin{eqnarray}\delta(x)&=&\lim_{\epsilon\to 0}\,\delta_\epsilon(x)\\&\equiv&
\lim_{\epsilon\to 0}\,\frac{1}{2\pi}\int_{-\infty}^{+\infty}\,e^{ikx-\epsilon k^2}\,dk \end{eqnarray}
The integral can be done explicitly by "completing the square''
\begin{eqnarray}-\epsilon k^2+ikx=-\epsilon
\left(k^2-\frac{ikx}{\epsilon}\right)
&=&-\epsilon\left[\left(k-\frac{ix}{2\epsilon}\right)^2-\left(\frac{ix}{
2\epsilon}\right)^2
\right]\\
&=&-\epsilon K^2-\frac{x^2}{4\epsilon} \end{eqnarray}
where $K=k-ikx/(2\epsilon)$. The integration variable can be changed from $k$ to $K$ and the integral evaluated
\begin{eqnarray}\delta_\epsilon(x)&=&\frac{1}{2\pi}\int_{-\infty}^{+\infty}\,e^{ikx-\epsilon k^2}\,dk\\
&=&\frac{e^{-x^2/4\epsilon}}{2\pi}\int_{-\infty}^{+\infty}\,e^{-\epsilon
K^2}\,dK\\
&=&\frac{e^{-x^2/4\epsilon}}{2\sqrt{\pi\epsilon}}\end{eqnarray}
These functions give us another insight into the generalized functions like the delta function. For each finite value of the positive number $\epsilon$ the function is a gaussian curve with a width proportional to $\sqrt{\epsilon}$ and a height proportional to $1/\sqrt{\epsilon}$. The "area under the curve'', that is $\int\delta(x)dx$  is always unity, for any $\epsilon$. As $\epsilon\to 0$, the functions  $\delta_{\epsilon}$  become more and more narrow and the peak higher and higher at $x=0$. The Dirac delta function  is the "singular limit'' of such a sequence of functions.