CMI CM-I Problem Sheet 12 (2012)

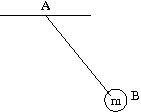
56. A mass m is attached to a massless spring (spring constant K, unstretched length L) and is free to oscillate in a vertical plane about A. Write the Lagrangian and the Hamiltonian of the system. Write both the Euler-Lagrange equation and the Hamilton's equation and show that they are identical.

57. Let the infinitesimal distance in a curved space be given by  $$ ds^2\ =\ ( g_{ab}(q_1,q_2,\ldots,q_N))d\dot{q}_a d\dot{q}_b\, dt^2\,=\, T^2 dt^2$$ where the summation over a and b is implied and take values 1,2,$\ldots$,N and $t$ is a parameter specifying the trajectory. Find the geodesic equation by minimising $ F(T)\,=\,T^2$ - this is the same as minimising $T$ as $T^2$ is a monotonic function of $T$. This saves considerable algebra.
Show that the geodesic equation is $$ \ddot{q}_p\,=\,\frac{1}{2}g^{-1}_{pa}[\frac{\partial g_{cd}}{\partial q_a}\,-\,\frac{\partial g_{ad}}{\partial q_c}\,-\,\frac{\partial g_{ac}}{\partial q_d}]d\dot{q}_cd\dot{q}_d $$ (again the summation convention has been used).

58. A particle of mass m moves on a spiral $x_3\,=\, k\,\theta, \rho\,=\, R $ ( we are using cylindrical co-ordinates $(\rho ,\theta ,x_3)$ , $x_3 $ being the vertical direction).Find the Hamiltonian and solve the equations of motion.

59. Consider the Lagrangian of a charged particle ( mass m , charge q) in the presence of an  electric $\vec{E}(\vec{r},t)$  and a magnetic field $\vec{B}(\vec{r},t)$. The Lagrangian for the particle is given by
$$ L\ =\ \frac{m\,\vec{\dot{r}}.\vec{\dot{r}}}{2}\,+\, q(-\phi(\vec{r},t)\,+ \vec{A}(\vec{\dot{r}},t)) $$ $$ \vec{E}(\vec{r},t)\ =\ -\vec{\nabla}\,\phi(\vec{r},t) -\frac{\partial \vec{A}(\vec{r},t)}{\partial t} $$ and $$ \vec{B}(\vec{r},t)\ =\ \vec{\nabla}\,\times\, \vec{A}(\vec{r},t) $$
Show that this leads to the correct force law $$ m\frac{d^2\vec{r}}{dt^2}\ =\ q(\vec{E}(\vec{r},t)\,+\,\vec{\dot{r}}\,\times\, \vec{B}(\vec{r},t)) $$ where $\vec{v}\,=\frac{d\vec{r}}{dt}$ is the velocity of the particle.

60. Consider a particle of mass m moving in a plane under the central force $$ F(r)\hat{r}\,=\,(-\frac{k}{r^2}\,+\,\frac{k'}{r^3})\hat{r} $$ with $k\,>\,0$.

1. Write the Lagrangian in terms of polar coordinates $(r,\theta) $.
2. Find the constants of motion.
3. Assume the angular momentum $l^2$ is greater than $-m\,k'$. Obtain an equation for $u\,=\,1/r$ as a function of $ \theta $. Get the general solution for $r$ as a function of $\theta $.