CHENNAI MATHEMATICAL INSTITUTE
CLASSICAL MECHANICS I
PROBLEM SHEET I
6th/7th August 2012
17th August 2012
Dimensional analysis is a useful tool in obtaining relations between physical quantities. Ofcourse the relation is only upto a numerical constant. Thus we know for a simple pendulum
$$
\omega =(\frac{g}{l} )^{1/2} \label{101}
$$
where $\omega$ is the angular frequency, related to the time period by $ T= 2 \pi/\omega$. ( Note in this case the result is exact!)We will consider two problems using dimensional analysis which also gives a simple insight into complex systems. It is necessary to pickup the right physical quantities relevent to the problem. (The numerical constant has to be chosen - first guess is 1). Three examples are provided as problems
1. An electric dipole of strength $P$ produces radiation when it oscillates with an angular frequency $\omega$. Here we are considering the dipole as an one dimensional oscillator. Using the fact that the energy density in an electric field $E$ is proportional to $\epsilon_0 E^2$, find the power radiated by the oscillator using dimensional analysis.
2.Consider a binary star system radiating gravitational energy because of its motion around each other in a circular of radius $R$ about their centre of mass. First, prove the dipole moment for mass vanishes about the centre of mass. However the next higher moment ( quadrupole) does not vanish which is defined as $\sum_{i=1}^2m_ir_i^2$ where $m_i$ is the mass located ar $\vec{r}_i$,measured from the centre of mass.Then use the relevent physical variable to write an expression for the power emitted by the system.
3. There is a famous analysis of G.I.Taylor, a British scientist who obtained the energy liberated by an atom bomb detonated ( referred to as Trinity) by studying pictures from Life magazine, which had published the mushroom developing at different times. This was obtained by using dimensional analysis and an ingenious choice of variables. ( This was done in late 1940's when the enrgy released was still a classified information). This has been converted into a problem (with hints)
Consider an explosion releasing a large amount of energy. Assume the shock wave produced in the atmosphere is hemispherical. Find the radius $R$ as a funtion of the time $t$ after explosion in terms of the energy $E$ released and other physical quantities relevent to the atmosphere ( atmospheric density and atmospheric pressure) and use dimensional analysis. You will find that here are two independent dimensionless variable in the problem -make a judicious choice and use the fact theat energy released is large and the time in which the shockwave expands is very small.
The part of the data from the life magazine is as follows:
| time(milliseconds) | radius of shockwave(metres) |
| 1.08 | 38.9 |
| 3.26 | 59.0 |
| 62 | 185 |
From this Taylor deduced that the energy released was of the order of $8\times 10^{13}J
$, which was a classifed data at that time. Verify Taylors estimate.
4. A particle P can travel in two different paths from XY to ZW - one, XYAZW and the other XYBZW. The initial velocity is V and XY and ZW is in the same horizontal plane. Neglecting friction, which path will take less time ? Give reasons.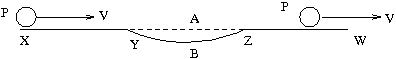
Earth's gravity acts in the vertical direction.
5. A person travels northward on an upward slope making an angle $30^0$ with the horizontal. Rain is falling towards east making an angle of $30^0$ with the vertical. The speed of the person is $U$ and that of rain is $V$. Find the angle the rain makes with the vertical as seen by the person.






 ||Message]
||Message]
